The simple structure of gases makes them readily adaptable to mathematical analysis from which has evolved a detailed theory of the behavior of gases. This is called the kinetic theory of gases. The theory assumes that a body of gas is composed of identical molecules which behave like minute elastic spheres, spaced relatively far apart and continuously in motion.
The degree of molecular motion is dependent upon the temperature of the gas. Since the molecules are continuously striking against each other and against the walls of the container, an increase in temperature with the resulting increase in molecular motion causes a corresponding increase in the number of collisions between the molecules. The increased number of collisions results in an increase in pressure because a greater number of molecules strike against the walls of the container in a given unit of time.
If the container were an open vessel, the gas would expand and overflow from the container. However, if the container is sealed and possesses elasticity, such as a rubber balloon, the increased pressure causes the container to expand. For instance, when making a long drive on a hot day, the pressure in the tires of an automobile increases, and a tire which appeared to be somewhat “soft” in cool morning temperature may appear normal at a higher midday temperature.
Such phenomena as these have been explained and set forth in the form of laws pertaining to gases and tend to support the kinetic theory.
Volume 1 × Pressure 1 = Volume 2 × Pressure 2
or
V1P1 = V2P2
Example: 10 ft3 of nitrogen is under a pressure of 500 psia. If the volume is reduced to 7 ft3, what will the new pressure be?[Figure 1]
V1P1 = V2P2
10 (500) = 7 (P2)
10 (500) ÷ 7 = P2
P2 = 714.29 psia
The useful applications of Boyle’s law are many and varied. Some applications more common to aviation are: (1) the carbon dioxide (CO2) bottle used to inflate life rafts and life vests; (2) the compressed oxygen and the acetylene tanks used in welding; (3) the compressed air brakes and shock absorbers; and (4) the use of oxygen tanks for high altitude flying and emergency use.
As a formula, this law is shown as follows:
Volume 1 × Absolute Temperature 2 = Volume 2 × Absolute Temperature 1
or
V1T2 =V2T1
Charles’ law also works if the volume is held constant, and pressure and temperature are the variables. In this case, the formula would be as follows:
P1T2 = P2T1
For this second formula, pressure and temperature must be in the absolute.
Example: A 15-ft3 cylinder of oxygen is at a temperature of 70 °F and a pressure of 750 psig. The cylinder is placed in the sun and the temperature of the oxygen increases to 140 °F.
What would be the new pressure in psig?
70 degrees Fahrenheit = 530 degrees Rankine
140 degrees Fahrenheit = 600 degrees Rankine
750 psig + 14.7 = 764.7 psia
P1T2 = P2T1
764.7 (600) = P2 (530)
P2 = 764.7 (600) ÷ 530
P2 = 865.7 psia
P2 = 851 psig
Pressure 1 (Volume 1) = Pressure 2 (Volume 2)
Temperature 1 Temperature
or
P1 (V1) (T2) = P2 (V2) (T1)
When using the general gas law formula, temperature and pressure must be in the absolute.
Example: 20 ft3 of the gas argon is compressed to 15 ft3. The gas starts out at a temperature of 60 °F and a pressure of 1,000 psig. After being compressed, its temperature is 90 °F. What would its new pressure be in psig?
60 degrees Fahrenheit = 520 degrees Rankine
90 degrees Fahrenheit = 550 degrees Rankine
1,000 psig + 14.7 = 1,014.7 psia
P1 (V1) (T2) = P2 (V2) (T1)
1,014.7 (20) (550) = P2 (15) (520)
P2 = 1,431 psia
P2 = 1,416.3 psig
RELATED POSTS
The degree of molecular motion is dependent upon the temperature of the gas. Since the molecules are continuously striking against each other and against the walls of the container, an increase in temperature with the resulting increase in molecular motion causes a corresponding increase in the number of collisions between the molecules. The increased number of collisions results in an increase in pressure because a greater number of molecules strike against the walls of the container in a given unit of time.
If the container were an open vessel, the gas would expand and overflow from the container. However, if the container is sealed and possesses elasticity, such as a rubber balloon, the increased pressure causes the container to expand. For instance, when making a long drive on a hot day, the pressure in the tires of an automobile increases, and a tire which appeared to be somewhat “soft” in cool morning temperature may appear normal at a higher midday temperature.
Such phenomena as these have been explained and set forth in the form of laws pertaining to gases and tend to support the kinetic theory.
Boyle’s Law
As previously stated, compressibility is an outstanding characteristic of gases. The English scientist, Robert Boyle, was among the first to study this characteristic that he called the “springiness of air.” By direct measurement he discovered that when the temperature of a combined sample of gas was kept constant and the absolute pressure doubled, the volume was reduced to half the former value. As the applied absolute pressure was decreased, the resulting volume increased. From these observations, he concluded that for a constant temperature the product of the volume and absolute pressure of an enclosed gas remains constant. Boyle’s law is normally stated: “The volume of an enclosed dry gas varies inversely with its absolute pressure, provided the temperature remains constant.” The following formula is used for Boyle’s law calculations. Remember, pressure needs to be in the absolute.Volume 1 × Pressure 1 = Volume 2 × Pressure 2
or
V1P1 = V2P2
Example: 10 ft3 of nitrogen is under a pressure of 500 psia. If the volume is reduced to 7 ft3, what will the new pressure be?[Figure 1]
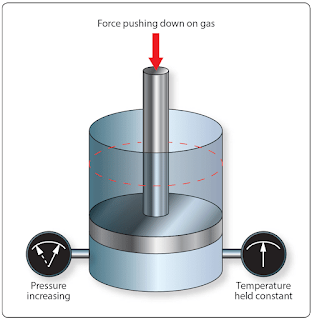 |
| Figure 1. Boyle’s law example |
V1P1 = V2P2
10 (500) = 7 (P2)
10 (500) ÷ 7 = P2
P2 = 714.29 psia
The useful applications of Boyle’s law are many and varied. Some applications more common to aviation are: (1) the carbon dioxide (CO2) bottle used to inflate life rafts and life vests; (2) the compressed oxygen and the acetylene tanks used in welding; (3) the compressed air brakes and shock absorbers; and (4) the use of oxygen tanks for high altitude flying and emergency use.
Charles’ Law
The French scientist, Jacques Charles, provided much of the foundation for the modern kinetic theory of gases. He found that all gases expand and contract in direct proportion to the change in the absolute temperature, provided the pressure is held constant. [Figure 2] |
| Figure 2. Charles’ Law example |
As a formula, this law is shown as follows:
Volume 1 × Absolute Temperature 2 = Volume 2 × Absolute Temperature 1
or
V1T2 =V2T1
Charles’ law also works if the volume is held constant, and pressure and temperature are the variables. In this case, the formula would be as follows:
P1T2 = P2T1
For this second formula, pressure and temperature must be in the absolute.
Example: A 15-ft3 cylinder of oxygen is at a temperature of 70 °F and a pressure of 750 psig. The cylinder is placed in the sun and the temperature of the oxygen increases to 140 °F.
What would be the new pressure in psig?
70 degrees Fahrenheit = 530 degrees Rankine
140 degrees Fahrenheit = 600 degrees Rankine
750 psig + 14.7 = 764.7 psia
P1T2 = P2T1
764.7 (600) = P2 (530)
P2 = 764.7 (600) ÷ 530
P2 = 865.7 psia
P2 = 851 psig
General Gas Law
By combining Boyle’s and Charles’ laws, a single expression can be derived which states all the information contained in both. The formula which is used to express the general gas law is as follows:Pressure 1 (Volume 1) = Pressure 2 (Volume 2)
Temperature 1 Temperature
or
P1 (V1) (T2) = P2 (V2) (T1)
When using the general gas law formula, temperature and pressure must be in the absolute.
Example: 20 ft3 of the gas argon is compressed to 15 ft3. The gas starts out at a temperature of 60 °F and a pressure of 1,000 psig. After being compressed, its temperature is 90 °F. What would its new pressure be in psig?
60 degrees Fahrenheit = 520 degrees Rankine
90 degrees Fahrenheit = 550 degrees Rankine
1,000 psig + 14.7 = 1,014.7 psia
P1 (V1) (T2) = P2 (V2) (T1)
1,014.7 (20) (550) = P2 (15) (520)
P2 = 1,431 psia
P2 = 1,416.3 psig
Dalton’s Law
If a mixture of two or more gases that do not combine chemically is placed in a container, each gas expands throughout the total space and the absolute pressure of each gas is reduced to a lower value, called its partial pressure. This reduction is in accordance with Boyle’s law. The pressure of the mixed gases is equal to the sum of the partial pressures. This fact was discovered by Dalton, an English physicist, and is set forth in Dalton’s law: “A mixture of several gases which do not react chemically exerts a pressure equal to the sum of the pressures which the several gases would exert separately if each were allowed to occupy the entire space alone at the given temperature.”RELATED POSTS
